May 24 2017
Materials categorized as “nanoporous” contain structures, that is, “frameworks,” whose pores have a diameter of nearly 100 nm. Such materials include various materials applied in different areas such as catalysis, gas separation and also medicine (e.g. activated charcoal).
The capacity of nanoporous materials relies on not only the chemical composition but also the shape of their pores. However, the shape of their pores cannot be evaluated easily. To date, Chemists depend on visual analysis to check whether the pores of two materials are similar. At present, Researchers from EPFL, belonging to the NCCR-MARVEL framework, have developed a new mathematical method that enables a computer to evaluate similarity of pore structures. The method enables searching of databases including innumerable nanoporous materials to find out new materials that have the optimal pore structure. The study has been published in the journal Nature Communications.
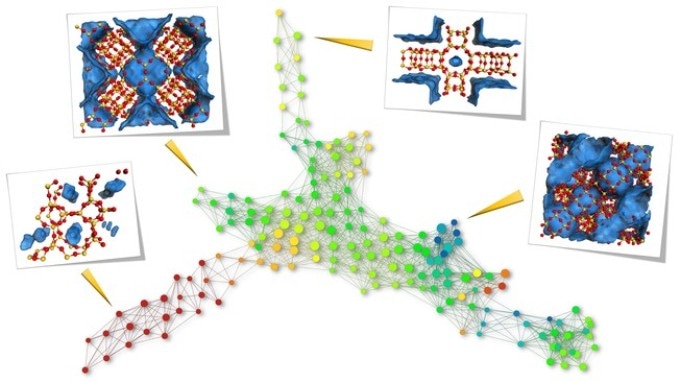Topological differences of top-performing materials for methane storage. Topological data analysis reveals the similarity between structures; each node represents a family of similar materials, while a network between two nodes indicates that they share at least one material. The further apart the nodes are, the more dissimilar the materials. The pictures show examples of nanoporous materials at the edges, and represent the topologically most different materials (red = Si, yellow = O, blue area = Pores). CREDIT: Berend Smit/EPFL. Figure obtained with the Ayasdi Core software platform.
The search for nanoporous materials
Although nanoporous materials fall into a wide class of materials and their chemical compositions differ greatly, the fact that connects them is the existence of nano-sized pores in their three-dimensional structure. These nano-sized pores are the reason behind the absorption and catalytic characteristics of these materials. The size of the pores can be 0.2-1000 nm. The shape and size, that is, the “geometry,” of the pose can have a definite impact on the characteristics of the material. As a matter of fact, the shape of the pores is a significant predictor of performance in terms of chemical composition.
At present, computers have the ability to produce large databases of promising materials and ascertain the materials with optimal performance for any given application, even before synthesizing the materials. However, the chemical composition of the materials is so adaptable that the number of probable new materials is nearly unlimited. Moreover there is no valid technique for quantifying and comparing the similarity of pore geometries. Overall, these factors point to the fact that determining the best nanoporous material for a given application is highly difficult.
Math to the rescue
A new method developed in a collaboration of the labs of Bernard Smit and Kathryn Bellward at EPFL uses a technique from applied mathematics called “persistent homology.” The technique has the ability to evaluate the similarity in the geometries of the pore structures by using mathematical tools generally used by Facebook and other social networking sites to find out similar faces in uploaded photos.
The persistent homology technique generates “fingerprints” that designate the shapes of the pores of each material in the database. Then, the fingerprints, which are represented by barcodes, are compared to estimate the similarity of the shapes of pores of the two materials, indicating that the technique can be applied to analyze databases and recognize materials that have identical pore structures.
The EPFL Researchers have demonstrated that the new technique is efficient in recognizing materials that have identical pore geometries. A category of nanoporous materials that will highly benefit from the research are zeolites and metal-organic frameworks (MOFs), which are used in gas separation, storage and catalysis.
As a case study, the Researchers used methane storage, which is an important aspect of renewable energy. The new technique demonstrated that nanoporous materials having a performance comparable to that of familiar top-performing materials can be discovered by searching databases for identical pore shapes.
On the contrary, the research demonstrates that the shapes of the pores of top-performing materials can be classified into topologically distinct categories and that materials from each class require a different optimization strategy.
We have a database of over 3,000,000 nanoporous materials, so finding similar structures through visual inspection is out of the question. In fact, going through the literature, we found that authors often don’t realize when a new MOF has the same pore structure as another one. So we really need a computational method. However, while humans are intuitively good at recognizing shapes as the same or different, we needed to work with the math department at EPFL to develop a formalism that can teach this skill to a computer.
Bernard Smit, École Polytechnique Fédérale de Lausanne
“In the field of algebraic topology, mathematicians have formulated the theory of persistence homology in any dimension,” stated Kathryn Hess. “Previous applications used only the first two of these dimensions, so it’s exciting that chemical engineers at EPFL have discovered a significant application that requires the third dimension as well.”
The study was possible due to a partnership between EPFL, INRIA (France) and UC Berkeley. The US Department of Energy, the National Center of Competence in Research (NCCR) “Materials’ Revolution: Computational Design and Discovery of Novel Materials (MARVEL),” the Deutsche Forschungsgemeinschaft (DFG), and the European Research Council (ERC; Horizon 2020; GUDHI) funded the study.