The major goal of quantum metrology has been to create highly entangled states with improved sensitivity per resource than classical approaches. However, collective observations are required to reach the maximum limitations in multi-parameter quantum information processing jobs, causing entanglement between different copies of the quantum state.
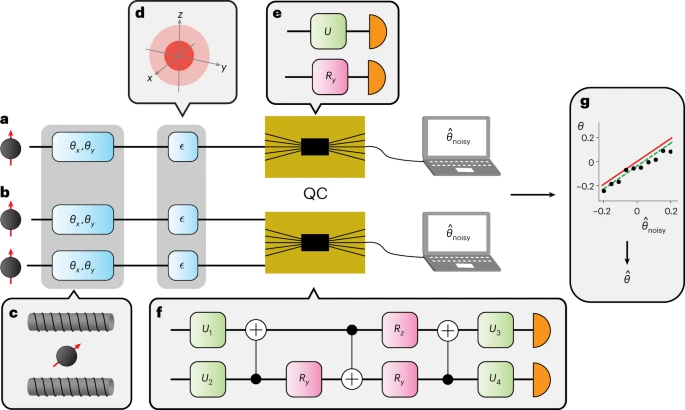
Experimental implementation of optimal collective measurements using quantum computers. a,b, Probe states are sent to the quantum computers (QC) individually for the single-copy measurement (a) and in pairs for the two-copy measurement (b). c,d, The qubit probes experience rotations, θx and θy, about the x and y axes of the Bloch sphere (c) before undergoing decoherence that has the effect of shrinking the Bloch vector (d). This rotation can be thought of as being caused by an external magnetic field that we wish to sense. e,f, The QCs then implement quantum circuits corresponding to the optimal single-copy (e) and two-copy (f) measurements. Two optimal single-copy circuits are shown, one for estimating θx and one for θy. g, Finally, error mitigation is used to improve the accuracy of the estimated angle. We create a model (green line) for how the noisy estimate of θ, θ^noisy (black dots), is related to the true value (red line). The model is then used to correct θ^noisy to produce the final estimate θ^θ^. Sample data from the F-IBM QS1 device downsampled by a factor of three are shown in g. Error bars are smaller than the markers. Credit: Nature Physics (2023). DOI: 10.1038/s41567-022-01875-7
A recent study published in Nature Physics focuses on this problem by describing a novel method for achieving accurate measurements of microscopic objects using quantum computers. This breakthrough could have practical applications in a wide range of next-generation technologies, including biomedical sensing.
Potential of Entanglement in Quantum Mechanics
Quantum mechanics, the branch of physics that deals with the behavior of matter and energy at a subatomic level, holds great promise for enhancing metrology and communications. A fundamental feature of quantum mechanics is entanglement, which has been the focus of much research in quantum metrology.
Entanglement is a property where two quantum systems are correlated in such a way that the state of one system cannot be described independently of the other system. These properties are known as conjugate characteristics.
This is a clear expression of Heisenberg's renowned uncertainty principle, which states that it is not feasible to concurrently assess two conjugate attributes of a quantum object with reasonable precision.
Entanglement plays a crucial role in quantum communication and quantum computation. However, there is a need to find new ways to generate and manipulate entanglement to fully harness the potential of quantum mechanics in these fields.
Challenges in Collective Measurements for Quantum Metrology
Quantum metrology aims to improve the precision and resource efficiency of measurements using quantum states. However, the current focus of quantum metrology has been on producing exceedingly entangled quantum states, which offer improved sensitivity per resource to classical methods.
Collective measurements, which generate entanglement between multiple copies of the quantum state, are necessary to reach the ultimate bounds in multi-parameter quantum metrology.
The Holevo bound is not reachable in most practical settings because it demands a collective measurement on an infinite number of copies of the quantum state. Contemporary approaches to collective measurements on optical systems are also limited in their scalability and the limited gate set available can make it harder to implement an arbitrary optimal measurement.
Scalability is a major challenge in quantum computing and quantum metrology. Moreover, the limited gate set accessible on optical systems can make it more difficult to incorporate an arbitrary optimal measurement. This is a significant limitation in the field, as it makes it difficult to implement large-scale quantum computing and quantum-enhanced sensing networks.
Highlights of the Current Study
In this study, the researchers employed theoretically ideal single- and two-copy collective measurements to estimate two non-commuting qubit rotations simultaneously.
"By entangling two identical quantum systems, we can acquire more information," co-author Dr. Syed Assad said. "There is some unavoidable noise associated with measuring any property of a quantum system. By entangling the two, we're able to reduce this noise and get a more accurate measurement."
The researchers implemented their optimal measurements on trapped-ion, superconducting, and photonic systems. In contrast to the limited gate set available on optical systems, this allows for a more flexible and scalable method for implementing collective measurements.
The Holevo Cramér–Rao bound (Holevo bound) was used as a theoretical framework for the design of the collective measurements. The Holevo bound sets the ultimate achievable limits in quantum multi-parameter approximation. By approaching this bound, the researchers were able to demonstrate that their measurements can achieve the ultimate attainable limits for multi-parameter estimation.
Findings and Future Perspective
The researchers found that their measurements allow for the implementation of quantum-enhanced sensing, even in high levels of decoherence, unlike the use of entangled probe states. The study also drew fundamental insights into the interpretation of the uncertainty principle.
This research has many physically motivated applications, including concurrently assessing phase and phase diffusion, quantum super-resolution, approximating the components of a three-dimensional field, and tracking chemical processes. Moreover, collective measurements enable quantum-enhanced detection even in significant decoherence.
The research also highlights the potential of superconducting, trapped-ion, and photonic systems to be used in large-scale quantum computing and quantum-enhanced sensing networks.
It is anticipated that the current approach will be more versatile and scalable for future research in the field and will pave the way for a new generation of quantum-enhanced sensing and communication technology.
Reference
Conlon, L. O. et al. (2023). Approaching optimal entangling collective measurements on quantum computing platforms. Nature Physics. Available at: https://doi.org/10.1038/s41567-022-01875-7
Disclaimer: The views expressed here are those of the author expressed in their private capacity and do not necessarily represent the views of AZoM.com Limited T/A AZoNetwork the owner and operator of this website. This disclaimer forms part of the Terms and conditions of use of this website.