Sep 18 2017
The rich behavior of two-dimensional (2D) materials, like electrons, molecules or atoms that are limited to move on a flat surface, was celebrated by the 2016 Nobel Prize in Physics.
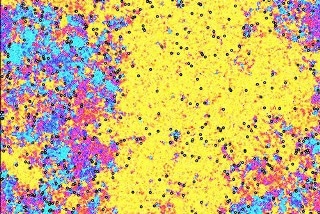 Snapshot of the hexatic phase in 2D hard disks. Credit: University of Bristol
Snapshot of the hexatic phase in 2D hard disks. Credit: University of Bristol
Such materials, when compared to their three dimensional (3D) counterparts, exhibit exotic and new properties, the explanation of which is at the cutting edge of condensed matter physics research.
The behavior of 2D crystals is considered to be an immensely interesting case. According to theoretical predictions, 2D crystals melt into a new phase called the hexatic, unlike 3D materials, which always melt into a liquid state or 'phase'.
In character, the hexatic is known to be intermediate between a crystal phase and a liquid, in that its constituent particles display only short ranged positional order (like a liquid) and long ranged orientational order (like a crystal).
It has been observed that the simplest 2D model material made up of identical hard disks also found it difficult to confirm that a two-dimensional crystal melts into a hexatic phase, which indeed was one of the longest standing problems in physics.
Following a number of attempts that were taken over four decades, it was only solved in 2011 by employing large scale computer simulations.
In a partnership between two GW4 universities, Dr John Russo from the University of Bristol's School of Mathematics and Professor Nigel Wilding, from the Department of Physics at the University of Bath, have incorporated the combined power of the high performance computers in both universities in order to demonstrate that the behavior of 2D crystals becomes more stranger when mixtures of two varieties of particles are considered.
On September 15th, 2017, their findings were published in the journal Physical Review Letters.
For this research we considered the 2D hard disk system studied previously, but with a twist: we introduced a second species of disk which is only 70 percent as big as the others. Interestingly we found that the presence of this second type of disk makes the hexatic phase disappear.
Dr John Russo, School of Mathematics, the University of Bristol
Professor Wilding added, "This occurs at surprisingly small concentrations of the small disks: swapping just one percent of the disks for the smaller species is enough to lose the hexatic."
We found that the hexatic is such a delicate state of matter because its entropy is only slightly greater than that of the liquid. Adding small particles raises the entropy of the liquid and this in turn destabilises the hexatic.
Professor Nigel Wilding, the Department of Physics, the University of Bath
The Researchers explain that their research contributes to the basic understanding of the captivating physics of matter in two dimensions, and also paves the way for designing new materials with exotic and strange properties.