Dec 12 2019
Over 150 years have passed since the publication of “A Dynamical Theory of the Electromagnetic Field” (1865) by James Clerk Maxwell. It is difficult to imagine lives without this publication, since this work has transformed the basic understanding of magnetic fields, electric fields, and light.
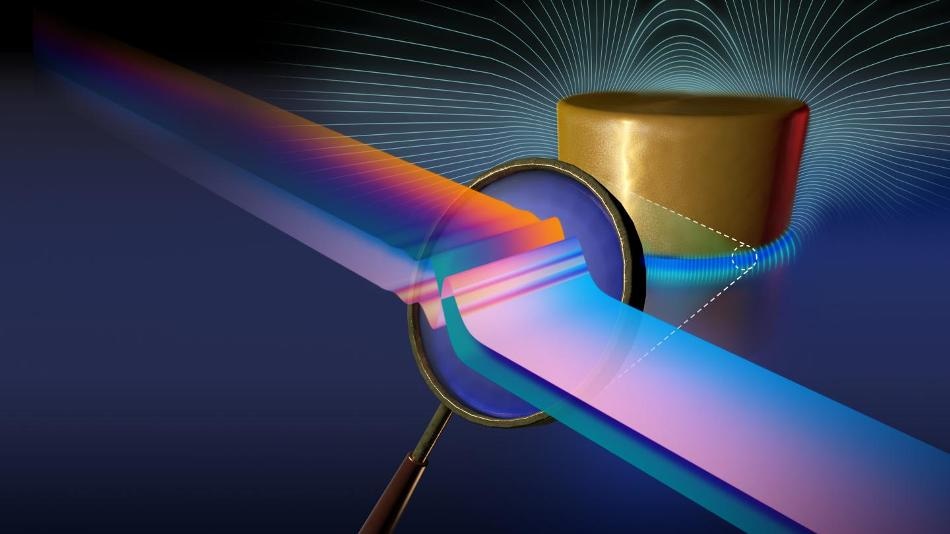This is an artistic illustration of nonclassical effects in nanoscale electromagnetism. When the confinement of electromagnetic fields in nanostructures becomes comparable to the electronic length scales in materials, the associated nonclassical effects can substantially affect the electromagnetic response. This illustration represents a film-coupled nanodisk (the nanostructure studied in this work); the insert in the magnifier shows the electronic length scales (in this case, the “thickness” of the surface-induced charge). Image Credit: Marin Soljaèi Research Group.
The 20 ingenious equations (at present elegantly reduced to four), the bulk electronic response functions (dielectric permittivity and magnetic permeability), and their boundary conditions at interfaces are the foundation of the ability of scientists to manipulate light and electromagnetic fields.
Hence, visualizing life without Maxwell’s equations is trying to picture life without most of present-day science, technology, and communications.
On macro (large) scales, the classical boundary conditions and bulk response functions are enough for explaining the electromagnetic response of materials, but for phenomena on smaller scales, nonclassical effects become significant.
A traditional treatment of classical electromagnetism does not explain the mere existence of effects like spill-out, nonlocality, and surface-enabled Landau damping. Why does this robust framework fail toward nanoscales?
This is because electronic length scales are at the center of nonclassical phenomena and are not part of the classical model. Electronic length scales can be regarded as the lattice spacing in solids or the Bohr radius: small scales that are meaningful for the available quantum effects.
At present, finally, there is a method to understand and model nanoscale electromagnetic phenomena. In the path-breaking Nature article titled “A General Theoretical and Experimental Framework for Nanoscale Electromagnetism”, Yang et al. have presented a model that can extend the validity of the macroscopic electromagnetism into the nano realm, thus bridging the scale gap.
Theoretical study has shown that their framework generalizes the boundary conditions by adding the electronic length scales in the form of so-called Feibelman d-parameters.
The role of d-parameters is similar to that of the permittivity, but for interfaces. In numerical modeling, each two-material interface is paired with corresponding Feibelman d-parameters and the Maxwell’s equations are solved using the new boundary conditions.
The authors of this experimental study investigated film-coupled nanoresonators, a typical multiscale architecture. The experimental setup was selected due to its nonclassical nature.
When we built our experiment, we were lucky enough to run into the right geometry that enabled us to observe the pronounced nonclassical features, which were actually unexpected and excited everyone. These features eventually enabled us to measure the d-parameters, which are hard to compute for some important plasmonic materials like gold (as in our case).
Yi Yang, Study Lead Author, Massachusetts Institute of Technology
Both the new model and experiments are of great importance for diverse applications and fundamental science. It makes a previously unexplored relationship between material science, electromagnetism, and condensed matter physics — potentially leading to further theoretical and experimental discoveries in all related areas, such as biology and chemistry.
With respect to applications, this research highlights the possibility of engineering the optical response beyond the classical regime — for example, to investigate the extraction of more power from emitters using antennas.
We expect this work to have substantial impact. The framework we present opens a new chapter for cutting-edge nanoplasmonics—the study of optical phenomena in the nanoscale vicinity of metal surfaces—and nanophotonics—the behavior of light on the nanometer scale—and for controlling the interaction of nanometer-scale objects with light.
Marin Soljacic, Professor, Massachusetts Institute of Technology
This study was partially supported by the Army Research Office through the Institute for Soldier Nanotechnologies under contract No. W911NF-18-2-0048 and W911NF-13-D-0001, and Air Force Office of Scientific Research (AFOSR) grant under contract No. FA9550-18-1-0436.
Y.Y. was partly supported by the MRSEC Program of the National Science Foundation under Grant No. DMR-1419807. D.Z. was supported by the National Science Scholarship from A*STAR, Singapore.
W.Y. was supported by Programme IdEx Bordeaux-LAPHIA (Grant No. ANR-10- IDEX-03-02) and project “Resonance” (Grant No. ANR-16-CE24-0013) of the French National Agency for Research (ANR). M.Z. was supported by the National Natural Science Foundation of China (Grant No. 11574078) the China Scholarship Council. T.C. was supported by the Danish Council for Independent Research (Grant No. DFF6108-00667).